matrix+ vector...

noob1988

matrix:










vector:
determine whether the line x=2-t  ,  y=3+4t  ,  z=-4-5t and the plane 3x-2y+7z+25=0
are parallel to each other.

怎样做？

noob1988

应该是找到solution了...

帮我看看对不对

n=(-1,4,-5)
v=(3,-2,7)

n.v=/=0
so, the plane are not parallel to the line?

antimatter

应该对瓜……………………

noob1988

应该对瓜……………………
antimatter 发表于 12-1-2010 10:51 PM

    刚刚放了matrix 的问题...
临时抱佛脚的我...
做pass year很多问题下

antimatter

刚放上去的都是很简单的问题而已。以你的聪明才智，我想应该不是问题吧……

noob1988

刚放上去的都是很简单的问题而已。以你的聪明才智，我想应该不是问题吧……
antimatter 发表于 13-1-2010 09:00 AM

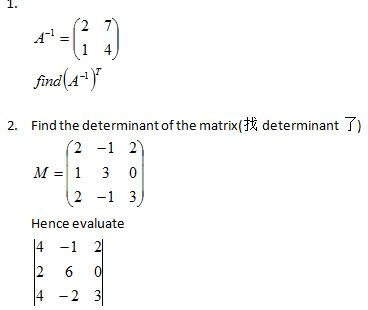
    evaluate的那个就是不懂...

antimatter

如果上面一排中间一个element 是-2 不是-1 的话，那么就是4×|M|。不然我想不到有什么其它的捷径了，你只好老老实实的算过那个matrix 的determinant 了。

noob1988

原来evaluate是要...找det?

antimatter

evaluate 的意思是叫你算出来，但是那个matrix 旁边的不是括弧，而是两条直线，这应该是代表determinant 吧。

noob1988

evaluate 的意思是叫你算出来，但是那个matrix 旁边的不是括弧，而是两条直线，这应该是代表determinant 吧 ...
antimatter 发表于 13-1-2010 02:36 PM

原来...假如是括弧呢？

antimatter

括弧不就是matrix 本身咯……

noob1988

evaluate 的意思是叫你算出来，但是那个matrix 旁边的不是括弧，而是两条直线，这应该是代表determinant 吧 ...
antimatter 发表于 13-1-2010 02:36 PM

    erm...我找determinant...答案和senior的不一样

他用
|4  -2  4|          |2  -1  2|
|2  6   0|     = 2|1   3  0|
|4  -2  6|          |2  -1  3|

2^3det(m)
2^3(7)=56

2我还可以理解
但^3怎样来的？

~HeBe~_@

他是用 formula  det(k A) = k^n det (A) where A is a nxn matrix.

Exp:  
      (4  -2  4)          (2  -1  2)
A = (2  6   0)     = 2(1   3  0)    = 2B
      (4  -2  6)          (2  -1  3)

det(A)= |A| = |2B| = det(2B) = 2^3  det(B).

为什么 ^3 ? 因为 matrix B 是 3x3 matrix.

回到问题，原有的问题是
Hence evaluate
|4  -1  2|     
|2  6   0|     
|4  -2  3|  

而不是 evaluate
|4  -2  4|          |2  -1  2|
|2  6   0|     = 2|1   3  0|
|4  -2  6|          |2  -1  3|

noob1988

他是用 formula  det(k A) = k^n det (A) where A is a nxn matrix.

Exp:  
      (4  -2  4)          ...
~HeBe~_@ 发表于 14-1-2010 09:50 AM

    所以说假如4*4他就k^4?
5*5就k^5
对吧？

~HeBe~_@

是的。。。。

noob1988

又有新问题了...

let p=(-1,2,1),q=(3,1,-1) and r=(2,0,4)be three vectors. find
i) a vertor tat is perpendicular to both p and q
ii) (q*r)-2p

antimatter

他是用 formula  det(k A) = k^n det (A) where A is a nxn matrix.

Exp:  
      (4  -2  4)          ...
~HeBe~_@ 发表于 14-1-2010 09:50 AM

    更fundamental 的方法应该是这样：

• If B results from A by interchanging two rows or columns, then det(B) = −det(A).
• If B results from A by multiplying one row or column with a number c, then det(B) = c · det(A).
• If B results from A by adding a multiple of one row to another row, or a multiple of one column to another column, then

注：这里是用第二个结果。
Reference: http://en.wikipedia.org/wiki/Determinant

antimatter

i) c(p × q), c is real number.
ii) This is simple and straight forward, i don't see why you can't do it yourself...

noob1988

i) c(p × q), c is real number.
ii) This is simple and straight forward, i don't see why you can't  ...
antimatter 发表于 14-1-2010 02:45 PM

做到了...原来是我粗心误把它当matrix...前面我*了些东西...
lol...

noob1988

更fundamental 的方法应该是这样：If B results from A by interchanging two rows or column ...
antimatter 发表于 14-1-2010 02:41 PM

   thx...